计算物理第九次作业

计算物理

---

摘要

我们将探究碰撞反射问题，研究小球在方形界面、球形界面中的运动轨迹，及其影响因素，同时本次作业就3.30题进行探究和解答

背景

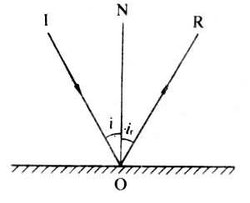
反射是在日常生活中较为常见的一种现象，无论是光的反射亦或是台球碰撞后的反弹，其遵循的基本规律都是反射定律，即反射角等于入射角。则对于速度，有如下的关系式：

$$\begin{eqnarray*} \vec{v}_{i,\perp}&=&(\vec{v}_i\cdot\hat{n})\hat{n}\\ \vec{v}_{i,\parallel}&=&\vec{v}_i-\vec{v}_{i,\perp}\\ \\ \vec{v}_{f,\perp}&=&-\vec{v}_{i,\perp}\\ \vec{v}_{f,\parallel}&=&\vec{v}_{i,\parallel} \end{eqnarray*}$$
同时我们知道，不同的边界条件将对反射的运动轨迹造成不同的影响，若为直线边界，则如图：

若为弧形边界，则类似Figure 3.19，初射角的分析将比之前略复杂：

当我们将圆形边界沿直径切开，并拉开一段距离（称之为 Stadium Billiard）,情况会复杂很多：

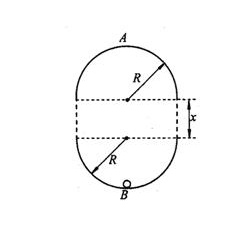
这里我们将研究不同边界条件对小球运动的影响

---

正文

1. 小球在方形边界中的运动

import pylab as pl
import math
class Billiard_Program :
    def __init__(self,i=0,initial_theta=math.pi/6,initial_velocity=1,total_time=500,\
                 initial_x=0.2,initial_y=0,time_step=0.01):
        self.theta=initial_theta
        self.v=initial_velocity
        self.v_x=[self.v*math.cos(self.theta)]
        self.v_y=[self.v*math.sin(self.theta)]
        self.x=[initial_x]
        self.y=[initial_y]
        self.time=total_time
        self.dt=time_step
        self.t=[0]
    def run(self):
        _time=0
        while(_time<self.time):
            self.x.append(self.x[-1]+self.v_x[-1]*self.dt)
            self.y.append(self.y[-1]+self.v_y[-1]*self.dt)
            if(self.x[-1]>1):
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(self.x[-1]>1):                
                        self.v_x.append(-self.v_x[-1])
                        break
            if(self.x[-1]<-1):
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(self.x[-1]<-1):                
                        self.v_x.append(-self.v_x[-1])
                        break
            if(self.y[-1]>1):
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(self.y[-1]>1):                
                        self.v_y.append(-self.v_y[-1])
                        break
            if(self.y[-1]<-1):
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(self.y[-1]<-1):                
                        self.v_y.append(-self.v_y[-1])
                        break
            self.t.append(_time)            
            _time += self.dt
    def show_results(self):
        pl.plot(self.x,self.y)
        pl.title('Billiard Program with $\\theta$=$\pi$/6')
        pl.xlabel('x')
        pl.ylabel('y')
        pl.xlim(-1,1)
        pl.ylim(-1,1)
        pl.legend()
        pl.show()
a = Billiard_Program()
a.run()
a.show_results()  

2. 小球在圆形界面中的运动

import pylab as pl
import math
class Billiard_Program :
    def __init__(self,i=0,initial_theta=math.pi/6,initial_velocity=1,total_time=80,\
                 initial_x=0.2,initial_y=0,time_step=0.01):
        self.theta=[initial_theta]
        self.v=[initial_velocity]        
        self.x=[initial_x]
        self.y=[initial_y]
        self.time=total_time
        self.dt=time_step
        self.t=[0]
        self.phi=[]
        self.v_x=[self.v[-1]*math.cos(self.theta[-1])]
        self.v_y=[self.v[-1]*math.sin(self.theta[-1])]
    def run(self):
        _time=0
        a=0.1
        r=1
        while(_time<self.time):
            self.x.append(self.x[-1]+self.v_x[-1]*self.dt)
            self.y.append(self.y[-1]+self.v_y[-1]*self.dt)
            if(self.y[-1]<-a*r and (pow(self.x[-1],2)+pow(self.y[-1]+a*r,2))>pow(r,2)):                
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(pow(self.x[-1],2)+pow(self.y[-1]+a*r,2)>pow(r,2)):  
                        cos_theta=self.x[-1]/r
                        sin_theta=(self.y[-1]+a*r)/r
                        vi_prependicular_x=abs(self.v_x[-1]*cos_theta+self.v_y[-1]*sin_theta)*cos_theta
                        vi_prependicular_y=abs(self.v_x[-1]*cos_theta+self.v_y[-1]*sin_theta)*sin_theta
                        vi_parallel_x=self.v_x[-1]-abs(self.v_x[-1]*cos_theta+self.v_y[-1]*sin_theta)*cos_theta
                        vi_parallel_y=self.v_y[-1]-abs(self.v_x[-1]*cos_theta+self.v_y[-1]*sin_theta)*sin_theta    
                        vf_parallel_x=vi_parallel_x
                        vf_parallel_y=vi_parallel_y
                        vf_prependicular_x=-vi_prependicular_x
                        vf_prependicular_y=-vi_prependicular_y
                        vf_x=vf_parallel_x+vf_prependicular_x
                        vf_y=vf_parallel_y+vf_prependicular_y
                        self.v_x.append(vf_x)
                        self.v_y.append(vf_y)
                        break
            if(self.y[-1]>a*r and (pow(self.x[-1],2)+pow(self.y[-1]-a*r,2))>pow(r,2)):                
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(pow(self.x[-1],2)+pow(self.y[-1]-a*r,2)>pow(r,2)):  
                        cos_theta=self.x[-1]/r
                        sin_theta=(self.y[-1]-a*r)/r
                        vi_prependicular_x=abs(self.v_x[-1]*cos_theta+self.v_y[-1]*sin_theta)*cos_theta
                        vi_prependicular_y=abs(self.v_x[-1]*cos_theta+self.v_y[-1]*sin_theta)*sin_theta
                        vi_parallel_x=self.v_x[-1]-abs(self.v_x[-1]*cos_theta+self.v_y[-1]*sin_theta)*cos_theta
                        vi_parallel_y=self.v_y[-1]-abs(self.v_x[-1]*cos_theta+self.v_y[-1]*sin_theta)*sin_theta    
                        vf_parallel_x=vi_parallel_x
                        vf_parallel_y=vi_parallel_y
                        vf_prependicular_x=-vi_prependicular_x
                        vf_prependicular_y=-vi_prependicular_y
                        vf_x=vf_parallel_x+vf_prependicular_x
                        vf_y=vf_parallel_y+vf_prependicular_y  
                        self.v_x.append(vf_x)
                        self.v_y.append(vf_y)
                        break
            if(-a<self.y[-1]<a and self.x[-1]>1):
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(self.x[-1]>1):                
                        self.v_x.append(-self.v_x[-1])
                        break
            if(-a<self.y[-1]<a and self.x[-1]<-1):
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(self.x[-1]<-1):                
                        self.v_x.append(-self.v_x[-1])
                        break
            self.t.append(_time)            
            _time += self.dt
    def show_results(self):
        pl.plot(self.x,self.y)
        pl.title('Circular stadium with $\\theta$=$\pi$/6')
        pl.xlabel('x')
        pl.ylabel('y')
        pl.xlim(-1.2,1.2)
        pl.ylim(-1.2,1.2)
        pl.legend()
        pl.show()
a = Billiard_Program()
a.run()
a.show_results()  

结论

1. 小球在方形界面中的运动

1.1 初始角度的影响

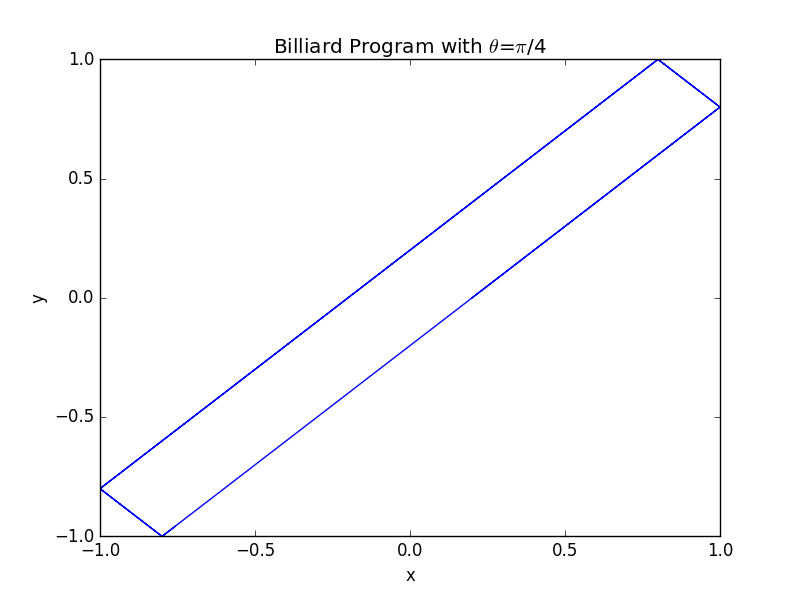
1.2 　以$\theta=\pi/6$ 为基础，增长程序运行时间


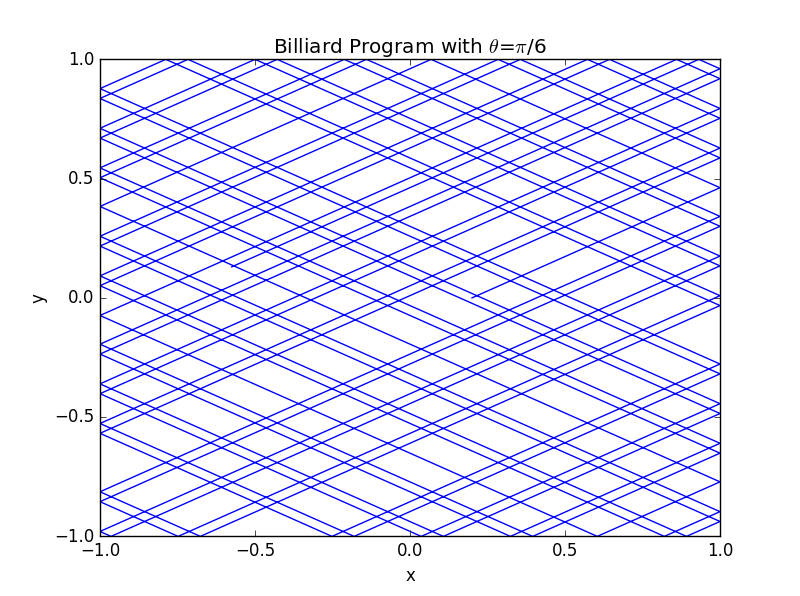
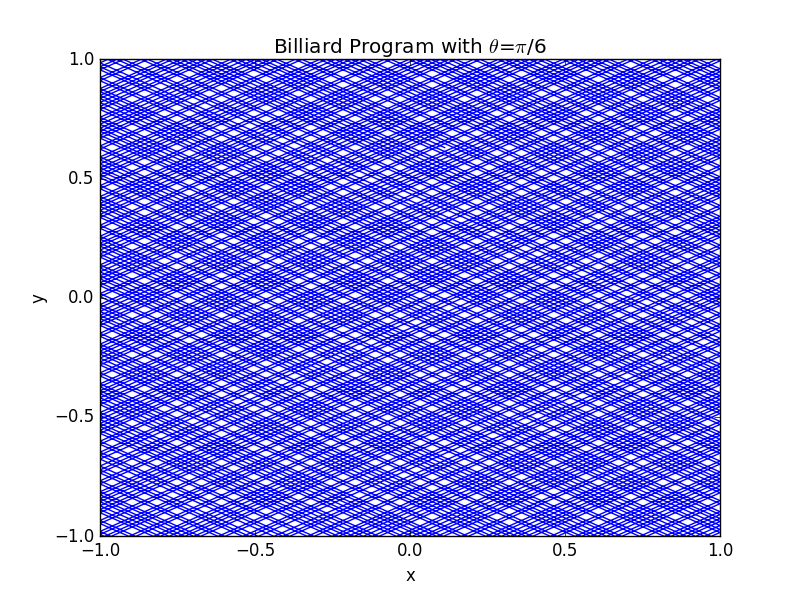
可见若时间足够长，轨迹将铺满整个平面

1.3　相空间

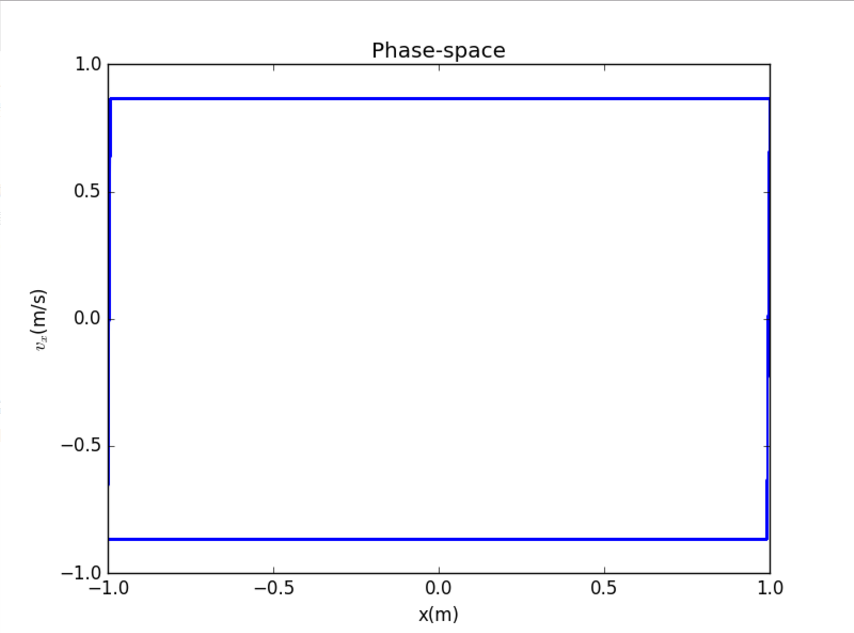
可见小球的速度在两确定值间变化

2. 圆形边界

2.1　正圆边界

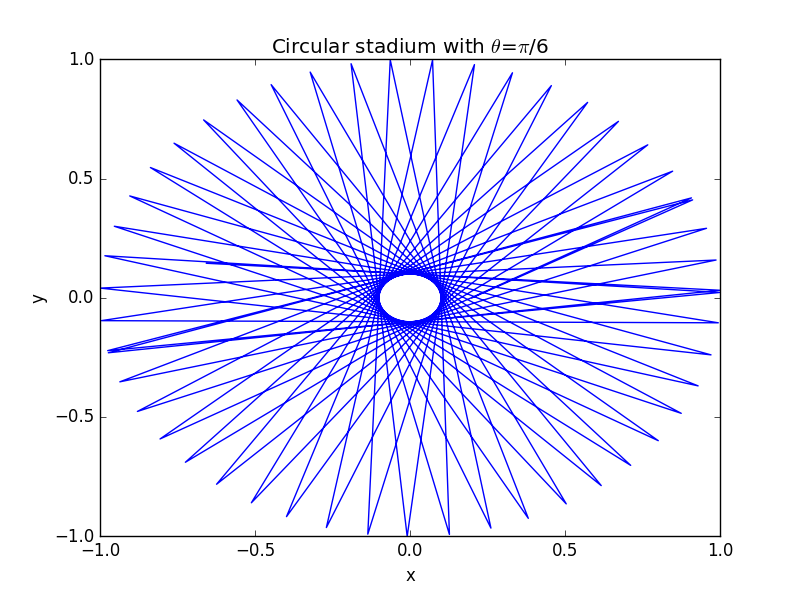
2.2　Stadium Billiard

改变$\alpha$的取值

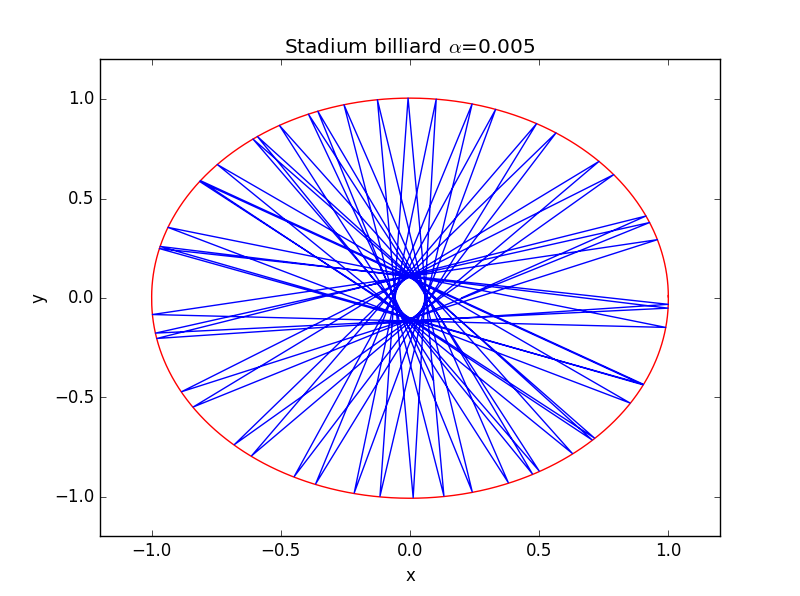
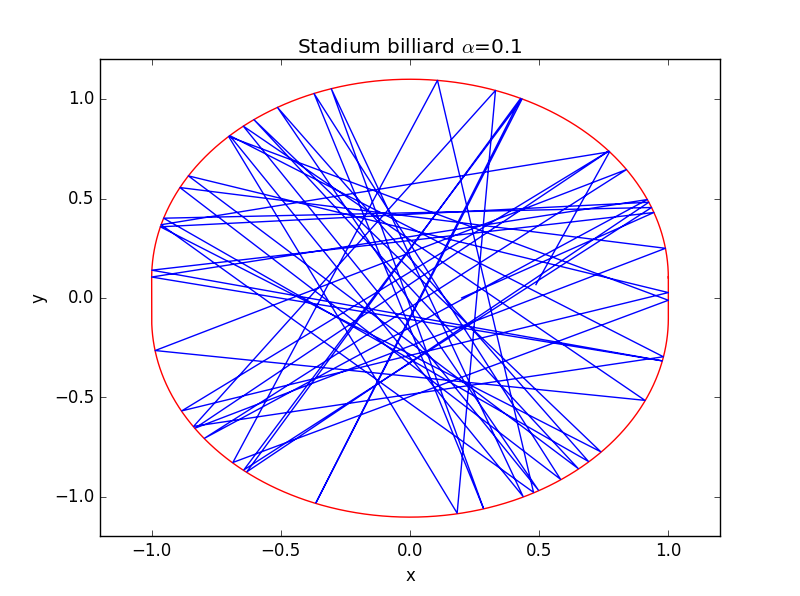
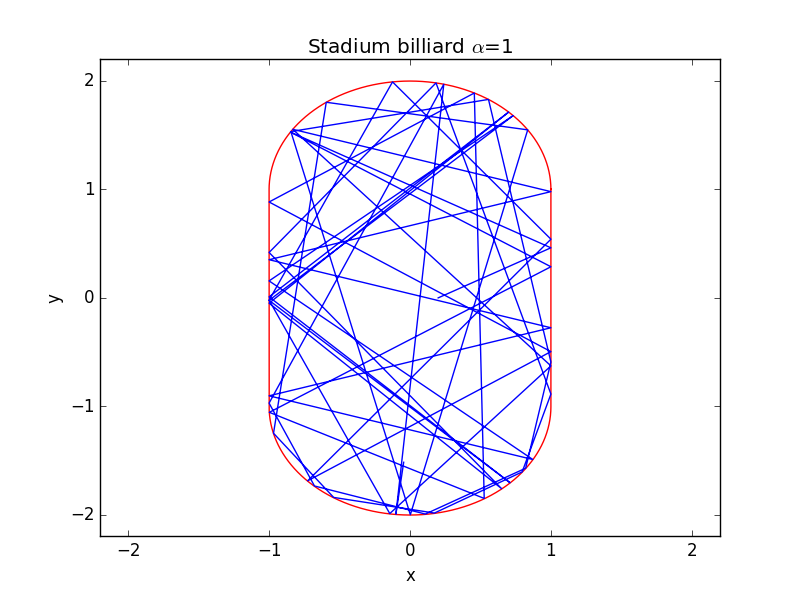
可见$\alpha=0.1$时整个运动轨迹已发生了巨大改变，若我们将$\alpha$改至足够大，使之完全成为“体育场形”则有：

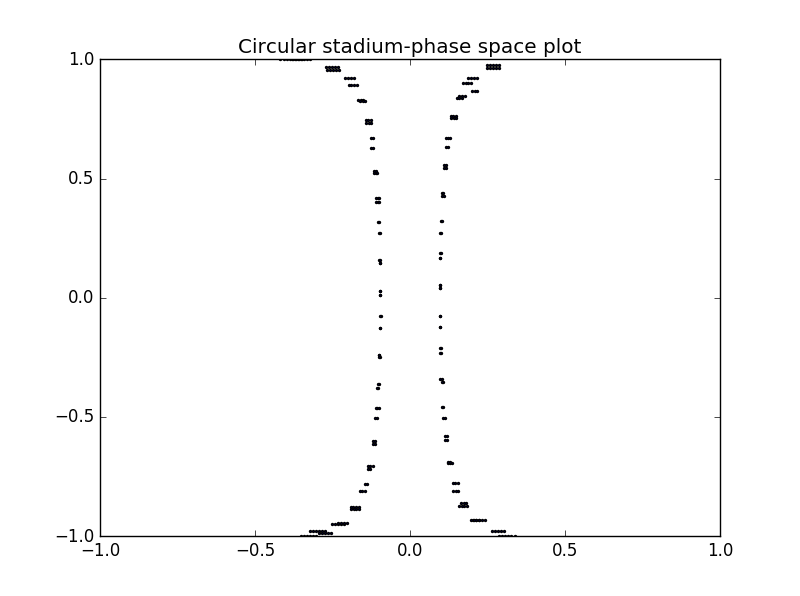
2.3 　相空间




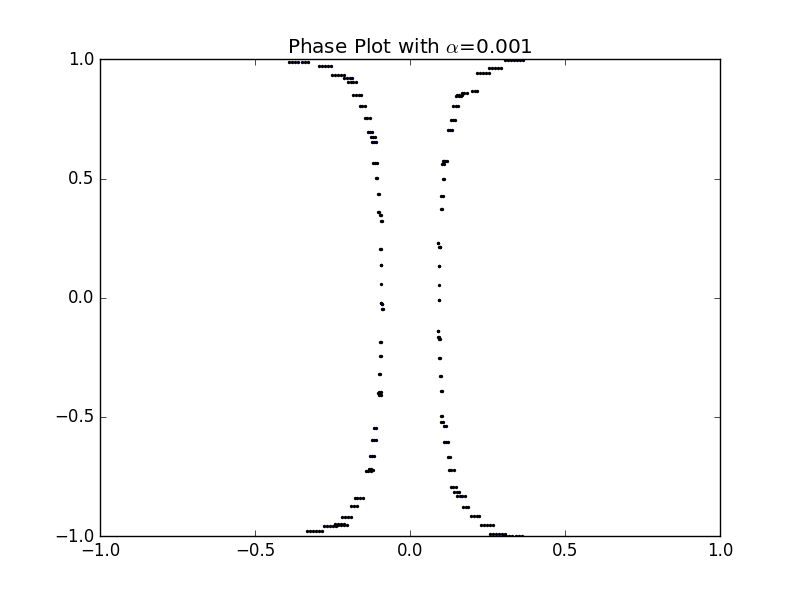
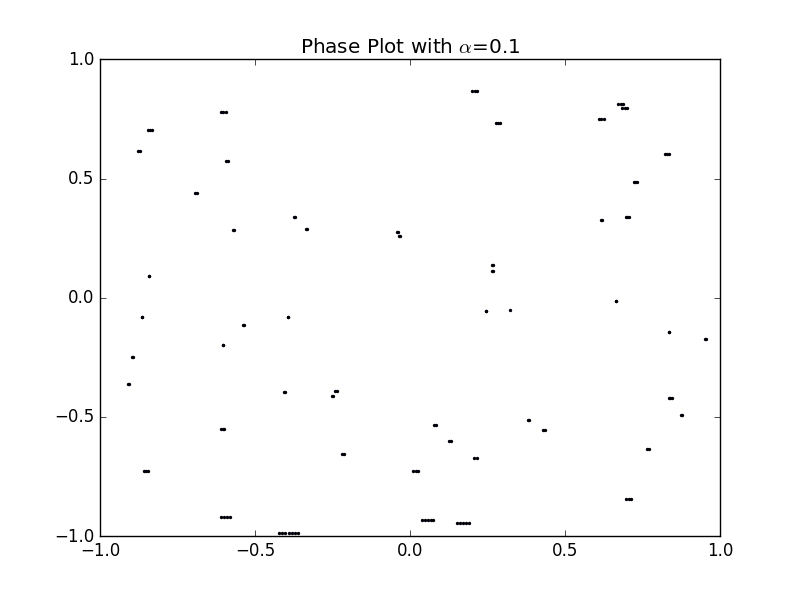
当$\alpha=0.1$时，轨迹已布满整个相空间，标志着运动以完全混乱

3 Problem 3.30

我们同时释放两个小球，他们有微小的初始位置差别，研究他们的运动，则有如下图像，此处设初始位置x相差0.001

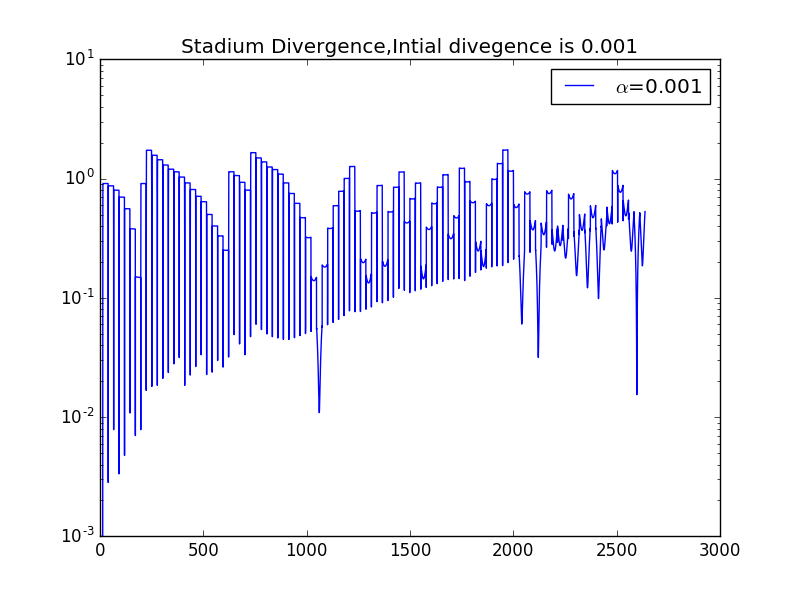
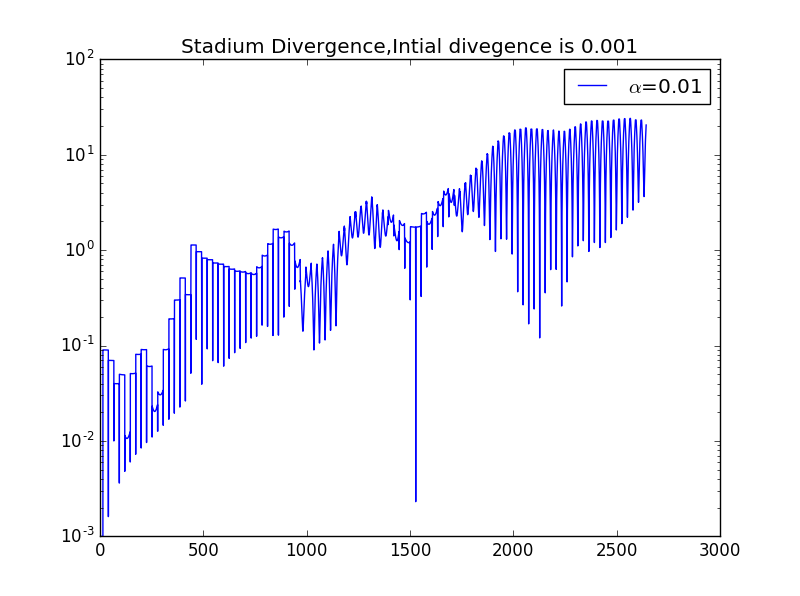
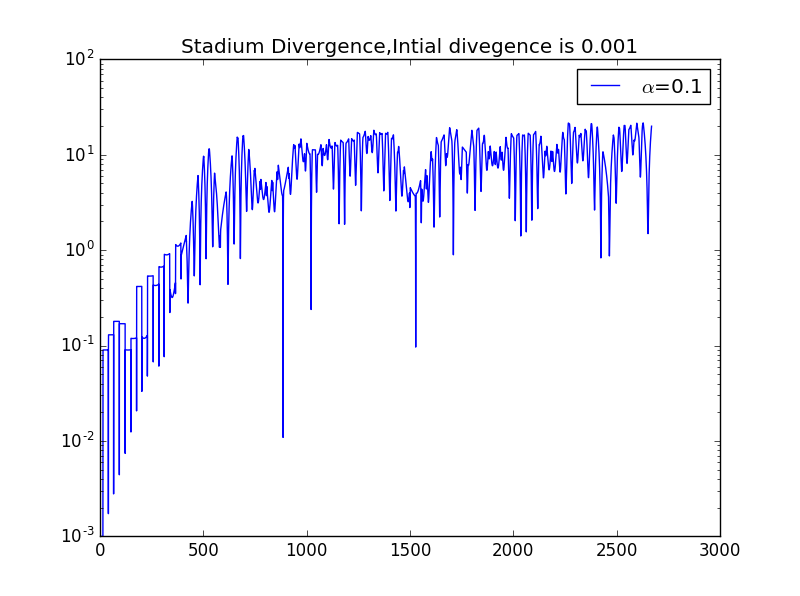
反思

1.　精度问题
为了提高小球在遇到边界时对边界的判断精度，我们采取如下算法，即超过边界时后退一步，再以更小步长前进，这一部分在程序中的表现如下：

if(self.x[-1]>1):
                self.x[-1]=self.x[-2]
                self.y[-1]=self.y[-2]
                while(1):                    
                    self.x.append(self.x[-1]+self.v_x[-1]*self.dt*0.01)
                    self.y.append(self.y[-1]+self.v_y[-1]*self.dt*0.01)
                    if(self.x[-1]>1):                
                        self.v_x.append(-self.v_x[-1])
                        break

当然，对于该问题同样可以一开始就采用小步长，但这样无疑会加大计算量，当程序越来越长时会严重拖慢计算速度，在本次作业的最终程序面前，我的计算机已经出现了卡顿，不得不缩减步长，若一开始就采用高精度，则结果一定是大大拖慢程序进程，因此对这种算法不予考虑
同时，我们可以检验程序的精度，即将出射点改为（0,0），出射角为$45^{\circ}$，若精度较高，则仅有一条直线，如图：

亦或进行局部放大，以球形边界为例（注意由于放大后出现失真，表现的似乎不满足反射定律）：

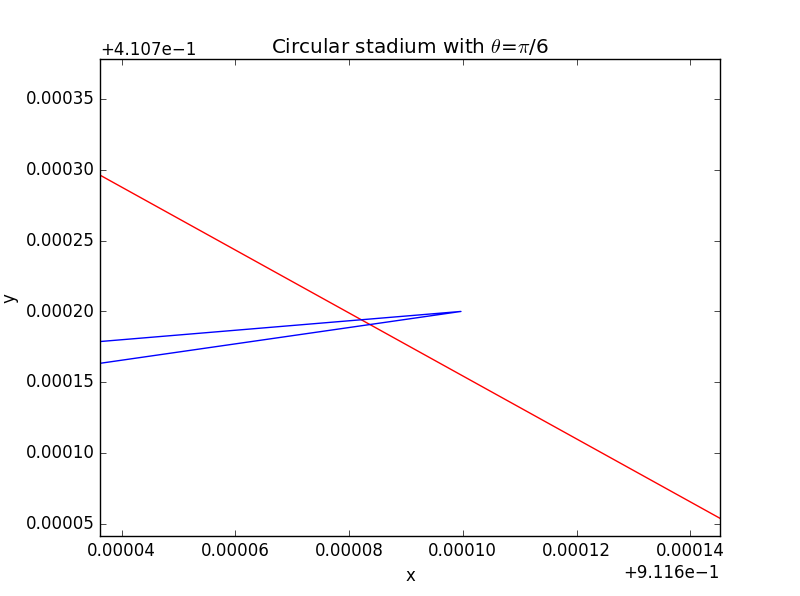
综上可见我们的精度还是比较高的

2. 程序报错
在编写过程中常会弹出此类错误：

此类错误非常容易出现，而产生的原因也很简单，是由于两变量字符串内所含元素的数量不相等，因此当给一个元素添加新的元素时，要注意另一坐标轴变量元素的添加

3. 进一步的探讨
其实我们还可以使用Vpython进行编写，从而更直观的看到小球的运动情况，
程序如下：

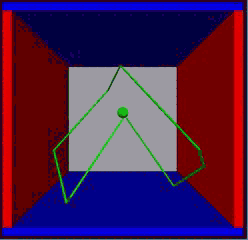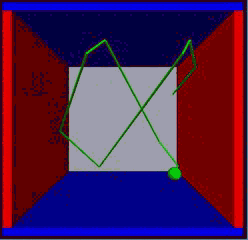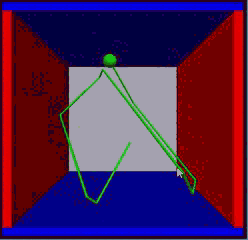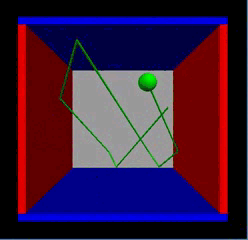
from visual import *
side = 4.0
thk = 0.3
s2 = 2*side - thk
s3 = 2*side + thk
wallR = box (pos=( side, 0, 0), size=(thk, s2, s3),  color = color.red)
wallL = box (pos=(-side, 0, 0), size=(thk, s2, s3),  color = color.red)
wallB = box (pos=(0, -side, 0), size=(s3, thk, s3),  color = color.blue)
wallT = box (pos=(0,  side, 0), size=(s3, thk, s3),  color = color.blue)
ball = sphere(pos=(0.2,0,0), color=color.red, radius = 0.4, make_trail=True, retain=200)
ball.trail_object.radius = 0.05
ball.v = vector(0.5,0.3,0)
side = side - thk*0.5 - ball.radius
dt = 0.5
t=0.0
while True:
  rate(100)
  t = t + dt
  ball.pos = ball.pos + ball.v*dt
  if not (side > ball.x > -side):
    ball.v.x = -ball.v.x
  if not (side > ball.y > -side):
    ball.v.y = -ball.v.y

结果如下图所示：

当然，我们也可让小球在三面上碰撞

鸣谢

张梓桐同学帮助纠正了程序在反射运动部分的小bug