Kefa and Park

time limit per test2 seconds  memory limit per test256 megabytes

树上相关 DFS

来源：
codeforces 580 C Kefa and Park
vjudge C Kefa and Park

Description

Kefa decided to celebrate his first big salary by going to the restaurant.

He lives by an unusual park. The park is a rooted tree consisting of n vertices with the root at vertex 1. Vertex 1 also contains Kefa's house. Unfortunaely for our hero, the park also contains cats. Kefa has already found out what are the vertices with cats in them.

The leaf vertices of the park contain restaurants. Kefa wants to choose a restaurant where he will go, but unfortunately he is very afraid of cats, so there is no way he will go to the restaurant if the path from the restaurant to his house contains more than m consecutive vertices with cats.

Your task is to help Kefa count the number of restaurants where he can go.

Input

The first line contains two integers, n and m (2 ≤ n ≤ 105, 1 ≤ m ≤ n) — the number of vertices of the tree and the maximum number of consecutive vertices with cats that is still ok for Kefa.

The second line contains n integers a1, a2, ..., an, where each ai either equals to 0 (then vertex i has no cat), or equals to 1 (then vertex i has a cat).

Next n - 1 lines contains the edges of the tree in the format "xi yi" (without the quotes) (1 ≤ xi, yi ≤ n, xi ≠ yi), where xi and yi are the vertices of the tree, connected by an edge.

It is guaranteed that the given set of edges specifies a tree.

Output

A single integer — the number of distinct leaves of a tree the path to which from Kefa's home contains at most m consecutive vertices with cats.

Examples

input

4 1
1 1 0 0
1 2
1 3
1 4

output

2

input

7 1
1 0 1 1 0 0 0
1 2
1 3
2 4
2 5
3 6
3 7

output

2

Note

Let us remind you that a tree is a connected graph on n vertices and n - 1 edge. A rooted tree is a tree with a special vertex called root. In a rooted tree among any two vertices connected by an edge, one vertex is a parent (the one closer to the root), and the other one is a child. A vertex is called a leaf, if it has no children.

Note to the first sample test: 

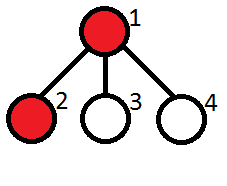
The vertices containing cats are marked red. The restaurants are at vertices 2, 3, 4. Kefa can't go only to the restaurant located at vertex 2.

Note to the second sample test: 

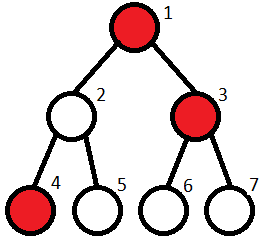
The restaurants are located at vertices 4, 5, 6, 7. Kefa can't go to restaurants 6, 7.

题意：

有一个人他发工资了，想要去吃顿好的，他住的那里就像一棵树一样，他住在一个点上，那个点上有餐馆，也可能有猫，由于他非常的怕猫，因此他去餐馆的时候碰到的猫不能超过m只，问你他最多能够去几家餐馆。
输入是这样的：第一行包含两个数：n和m，接下来的一行有n个数，表示这n个点有没有猫（有为1，无为0）；接下来的n-1行表示了每个点之间的关系
最后输出他最多能够去的餐馆数

解题思路：

处理的那些都是一样的，dfs就按照题来就是......但是有些我写来是错了，，，然后又改了

完整代码：

#include<stdio.h>
#include<string.h>
#include<math.h>
#include<vector>
#include<algorithm>
using namespace std;
vector<int>g[100010];
int num[100010];///数组
int vis[100010];///标记该点是否走过
int n,m;
int dfs(int u,int count)
{
    int ans=0;
    vis[u]=1;
    int flag=0;
    for(int i=0;i<g[u].size();i++)
    {
        int v=g[u][i];
        if(vis[v]==0)
        {
            flag=1;
            if(num[v]==1)
            {
                if(count<m)
                    ans+=dfs(v,count+1);///
            }
            else
                ans+=dfs(v,0);
        }
    }
    if(flag==0)
        return 1;
   return ans;
}
int main()
{
    while(scanf("%d%d",&n,&m)!=EOF)
    {
        memset(num,0,sizeof(num));
        memset(vis,0,sizeof(vis));
        for(int i=1;i<=n;i++)
          {
               scanf("%d",&num[i]);
               g[i].clear();
          }
        int a,b;
        for(int i=1;i<n;i++)
        {
            scanf("%d%d",&a,&b);
            g[a].push_back(b);
            g[b].push_back(a);
        }
        int ans=dfs(1,num[1]);
        printf("%d\n",ans);
    }
    return 0;
}